Express the series as a rational function – Expressing series as rational functions is a fundamental technique in mathematics, providing a powerful tool for representing complex functions as simpler algebraic expressions. This comprehensive guide delves into the concept of rational functions, exploring their properties, applications, and the methods used to express series as rational functions.
Rational functions, characterized by their quotient form, offer a versatile representation for functions with poles and zeros. Understanding their behavior and the techniques for expressing series as rational functions is crucial in various fields, including engineering, physics, and economics.
Rational Functions

A rational function is a function that can be expressed as the quotient of two polynomials. The general form of a rational function is:
$$f(x) = \fracp(x)q(x)$$
where p(x) and q(x) are polynomials.
Examples of rational functions include:
- $$f(x) = \fracx+1x-1$$
- $$f(x) = \fracx^2+1x-2$$
- $$f(x) = \frac1x^2+1$$
The poles of a rational function are the values of x for which the denominator q(x) is zero. The zeros of a rational function are the values of x for which the numerator p(x) is zero.
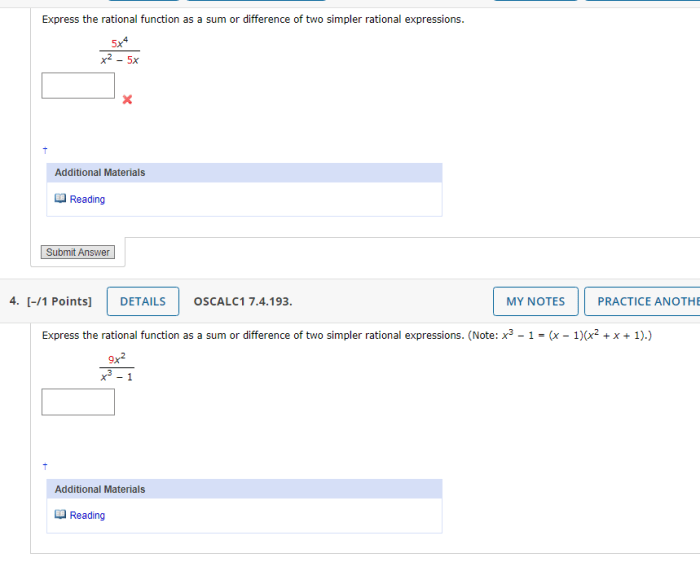
Expressing Series as Rational Functions
The method of partial fractions can be used to express a series as a rational function. The steps involved in applying partial fractions are as follows:
- Factor the denominator of the rational function.
- For each factor of the denominator, create a corresponding partial fraction.
- Solve for the constants in the partial fractions.
- Combine the partial fractions to obtain the rational function.
The different types of partial fractions and their corresponding denominators are:
- Linear factor:$$\fracAx+Bx-a$$
- Irreducible quadratic factor:$$\fracAx+Bx^2+bx+c$$
- Repeated linear factor:$$\fracA(x-a)^n$$
Applications of Rational Functions

Rational functions have a wide range of applications in various fields, including:
- Engineering:Rational functions are used to model the behavior of electrical circuits, mechanical systems, and fluid flow.
- Physics:Rational functions are used to describe the motion of objects, the propagation of waves, and the behavior of electromagnetic fields.
- Economics:Rational functions are used to model the behavior of markets, the growth of economies, and the distribution of income.
Here are some specific examples of how rational functions are used to solve real-world problems:
- Engineering:A rational function can be used to model the voltage across a capacitor in an electrical circuit.
- Physics:A rational function can be used to describe the trajectory of a projectile.
- Economics:A rational function can be used to model the demand for a product as a function of its price.
Extensions and Variations: Express The Series As A Rational Function
There are a number of extensions and variations of the method of partial fractions, including:
- Heaviside expansion:The Heaviside expansion is a method for expressing a rational function as a sum of exponential functions.
- Laplace transform:The Laplace transform is a method for converting a function of time into a function of a complex variable. The Laplace transform can be used to solve differential equations and integral equations.
These methods can be used to handle more complex rational functions that cannot be expressed using the method of partial fractions alone.
Commonly Asked Questions
What is the general form of a rational function?
A rational function is expressed as P(x)/Q(x), where P(x) and Q(x) are polynomials and Q(x) is not the zero polynomial.
How can I express a series as a rational function using partial fractions?
Partial fractions involve decomposing the rational function into simpler fractions with linear or quadratic denominators, which can then be integrated or differentiated as needed.
What are the applications of rational functions in real-world problems?
Rational functions are used in modeling physical phenomena, electrical circuits, economic systems, and other applications where functions with poles and zeros arise.